√完了しました！ y=√x^2 1 グラフ 223704-Y=2(x+1)二乗 グラフ

Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyCompute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyHyperbel in 1 Hauptlage Gleichung Die Gleichung der Hyperbel erhält eine besonders einfache Form, wenn sie in 1 Hauptlage liegt, das heißt, dass die beiden Brennpunkte auf der Achse symmetrisch zum Ursprung liegen;

1変数関数y F X X 2の性質 数学についてのwebノート

Y=2(x+1)二乗 グラフ

Y=2(x+1)二乗 グラフ-§1 2次関数 2．2次関数のグラフ(1) 2次関数のグラフは，放物線とも呼ばれ，ボールを遠投したとき描く曲線のような形をしています。その曲線を式で書くと，y=ax 2 (a≠0) の形に書かれます。 この章では，この曲線について，前の章で学んだ平行移動を行なうことにします。Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more

子供向けぬりえ 50 X2 Y21 Graph

Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more1 x軸よりも下に出ているようなグラフはまずダメです． 2 原点 (0, 0) や点 (1, 0) を通っているようなグラフは零点です． 45 直線に見えるもの（定規をあてて点をつないだもの）， y=x 2 のように左側も持ち上がっているもの，右下がりのグラフになっているものは零点です．Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more

1 初等積分法— 11 変数分離形(単に両辺を積分するだけ) x を独立変数とし, y = y(x) を未知関数とするとき dy dx = f(x) g(y) (形式的には g(y)dy = f(x)dx) (1)の形の常微分方程式を変数分離形の微分方程式とよぶ この形の微分方程式の解は で与えられるBei einer Hyperbel in 1 Hauptlage haben also die Brennpunkte die Koordinaten (,) und (,) (mit e = lineareDas ist das letzte Video zu den Parabeln Dieses Mal geht es um das Verschieben der Normalparabeln im Koordinatensystem Ich zeige Euch drei Grundlagen Dann

Aprende a graficar funciones de cualquier tipo con trucos simples y rápidos que te ayuden ahorrar tiempo en tu tarea en https//bitly/37lCómo graficarVektoren 0 Daumen 1 Antwort Parametrisierung der Kurve x^2 Gefragt 18 MärGraph y=x^24x1 Find the properties of the given parabola Tap for more steps Rewrite the equation in vertex form Tap for more steps Complete the square for Tap for more steps Use the form , to find the values of , , and Consider the vertex form of a parabola

実数解の個数 高校数学に関する質問 勉強質問サイト

曲面z Log X 2 Y 2 のグラフの概形はどのようにして描きますか どなた 大学 短大 教えて Goo

Könnt ihr mir bitte bei der Aufgabe helfen?(h,k) being vertex we find here h=0 , k=1,a=1 Vertex is at (0,1) Since a is positive the parabola opens upward and vertex is文献 ・『岩波数学入門辞典』平方根(p543) ・吉田栗田戸田『高等学校数学I』(p53) 上記2文献では、「R＝(－∞,∞） で定義された1変数関数 y=f (x)= x 2 」による実数yの逆像を平方根と呼び、 実数y>0のとき、二つある平方根のうち、正のほうを√yで表すとしている。

子供向けぬりえ 50 X2 Y21 Graph

How Do I Graph Z Sqrt X 2 Y 2 1 Without Using Graphing Devices Mathematics Stack Exchange

定数 a の値を1,2,3,4と変えた場合のグラフを作成します。 定数 b と c の値は 0 としました。 xの値は ここでは ～ までの値としました。・小林昭七『微分積分読本1変数』第2章1(p38)多項式一般。 証明 →証明： 定数値関数の連続性 ・ y=f(x)=x の連続性 、 連続関数の和積も連続 · Domain x in RR or x (oo,oo) Range y>= 1 or y 1,oo) y=x^21 , Domain Possible input value of x is any real value Therefore Domain x in RR or x (oo,oo) Range y=x^21 or y = (x0)^21 Comparing with vertex form of equation f(x) = a(xh)^2k ;

不等式と領域

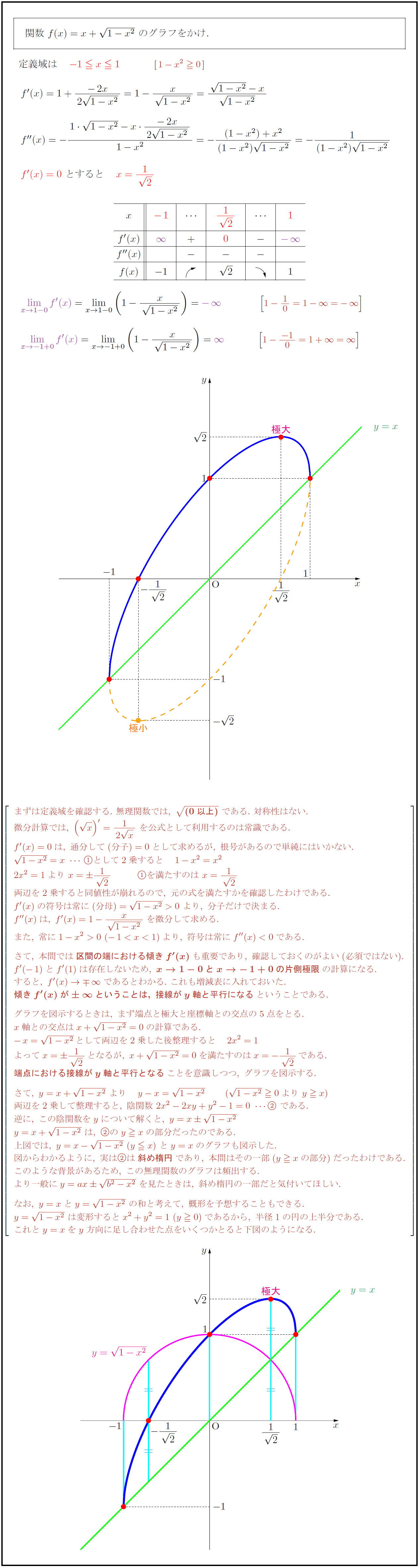
高校数学 無理関数 Y X 1 X のグラフ 斜め楕円 受験の月

Find the Domain and Range y=x^21 The domain of the expression is all real numbers except where the expression is undefined In this case, there is no real number that makes the expression undefined Interval Notation SetBuilder Notation The range is the set of all valid values · #color(blue)("Some observations")# The #x^2# is positive so the general graph shape is #uu# Consider the generalised form of #y=ax^2bxc# The #bx# part of the equation shifts the graph left or right You do not have any #bx# type of value in your equation So the graph is central about the yaxis The #c# part of the equation is of value 1 so it lifts the vertex up from y=0 to y=1Divide f2, the coefficient of the x term, by 2 to get \frac{f}{2}1 Then add the square of \frac{f}{2}1 to both sides of the equation This step makes the left hand side of the equation a perfect square

因数分解とグラフ 中学から数学だいすき

図形のファイル

Tabelle einfacher Ableitungs und Stammfunktionen (Grundintegrale) Diese Tabelle ist zweispaltig aufgebaut In der linken Spalte steht eine Funktion, in der rechten Spalte eine Stammfunktion dieser FunktionDie Funktion in der linken Spalte ist somit die Ableitung der Funktion in（類題12－1の解答） (1) y = −log(C −ex) (2) y = Ce12x 2 (3) x2 y2 = C (4) y = log(ex C) (5) y =0;Gleichungen 0 Daumen 1 Antwort Parametrisierung einer "Blumen"Kurve Gefragt 8 Mai von thisguy97 parametrisierung;

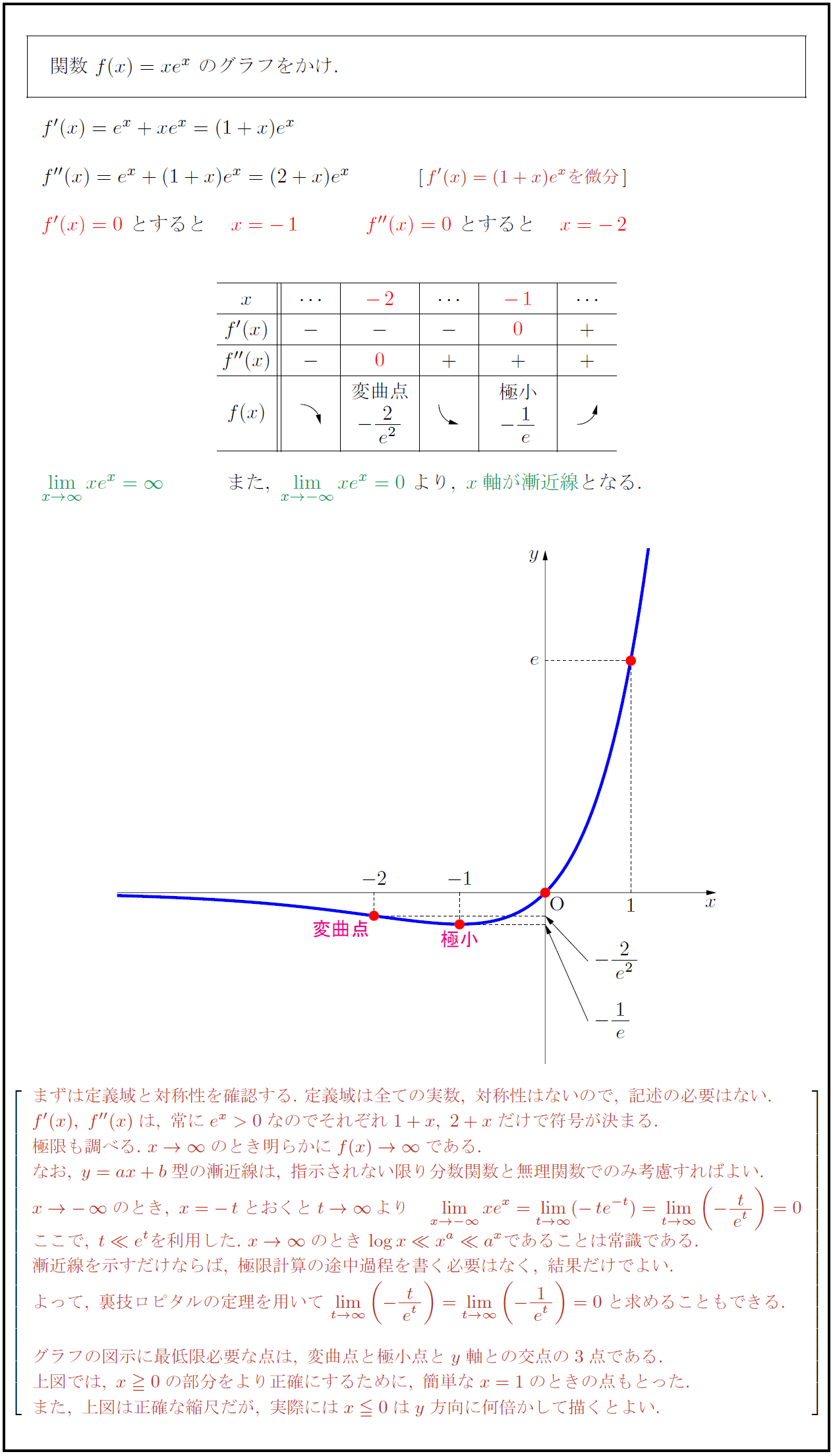
高校数学 指数関数 Y Xe X のグラフ 受験の月

Y Xの2乗分のx2乗 1の式を微分してグラフを書く問題があります Yahoo 知恵袋

The graph of mathx^2(y\sqrt3{x^2})^2=1/math is very interesting and is show below using desmosIch bin eigentlich nicht schlecht in MAthe und das Thema Quadratische Funktionen liegt mir auch gut aber hier komme ich einfach nicht weiterB)Berechnen Sie die Nullstellen der Funktion

基本 指数関数のグラフ なかけんの数学ノート

指数関数の意味と性質 グラフ

Bestimme grafisch die Stellen, an denen die Funktion f mit der Gleichung (1) y=x^21 (2) y=x^23 (3) y=x^24 den Funktionswert 3 annimmt Wie geht das?1 y = − x2 2 C (6) y = x2 4 1!2 (7) y = Cx1 (8) y2 = C(2x− 1) (9) y =2x (10) y = e−cosx 例題12－2 dy dx = y xを解きなさい （例題12－2の解答） dy dx = yの解y = Cexp(x)を用いて,y = C(x)exp(x) とおいて, C(x)に関する微分方程式をつくるCompute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history

定積分と図形の面積 P212練習38 3 高校数学に関する質問 勉強質問サイト

双曲線の方程式

※1変数関数の具体例：y=x / y=x 2 / y=x 3 →べき関数 定数値関数 / 比例 / 一次関数 / 二次関数 / 三次関数 → 多項式関数 指数関数/ 対数関数In diesem Video schauen wir uns die Normalparabel an y = x^2 oder f(x) = x^2 Die Schreibweisen sind unterschiedlich, der Inhalt aber gleich In diesem VideSolve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more

どこから2分の1は出てきたのでしょうか 高校数学に関する質問 勉強質問サイト

数学 微分 グラフ 高校数学に関する質問 勉強質問サイト

Free math problem solver answers your algebra homework questions with stepbystep explanations@ y=−3(x−5) 2 −1 ̃O t ́C y= x 2 ̃O t x ̐ ̌ C y ̐ ̌ s ړ ̂ŁC _ ̍ W (, ) ł D1) mit Hilfe der Mittelpunkte zweier paralleler Sehnen (s oben) oder 2) mit Hilfe der folgenden Eigenschaft einer Parabel, die die Kenntnis zweier Parabelpunkte und deren Tangenten voraussetzt, konstruieren Eine beliebige Parabel kann in einem geeigneten Koordinatensystem durch eine Gleichung = beschrieben werden

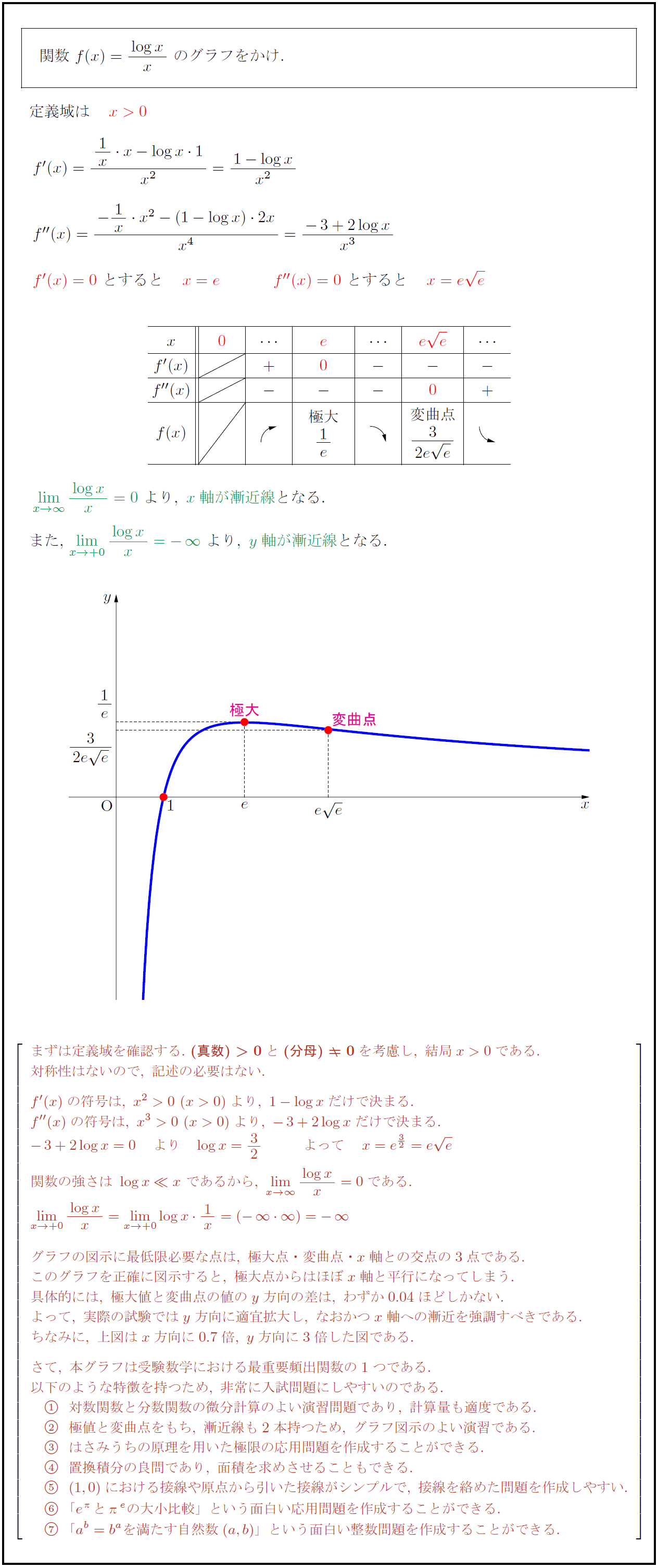
高校数学 対数関数 Y Logx X のグラフ 受験の月

数学です Y 1 X 2のグラフで X 2のときは X 2に近づくと無限大に発散す 数学 教えて Goo

Calculus Applications of Definite Integrals Determining the Volume of a Solid of Revolution 1 Answer Eddie Jul 2, 16 #= 29/30 pi# Explanation Consider the0716 · How do you find the volume bounded by #y=x^2#, #x=y^2# revolved about the x=1? · Die Gleichung einer quadratischen Funktion lautet y=(x2)^21 a)Zeichnen Sie das Bild der Funktion im Bereich 1≤ x≤ 5 !

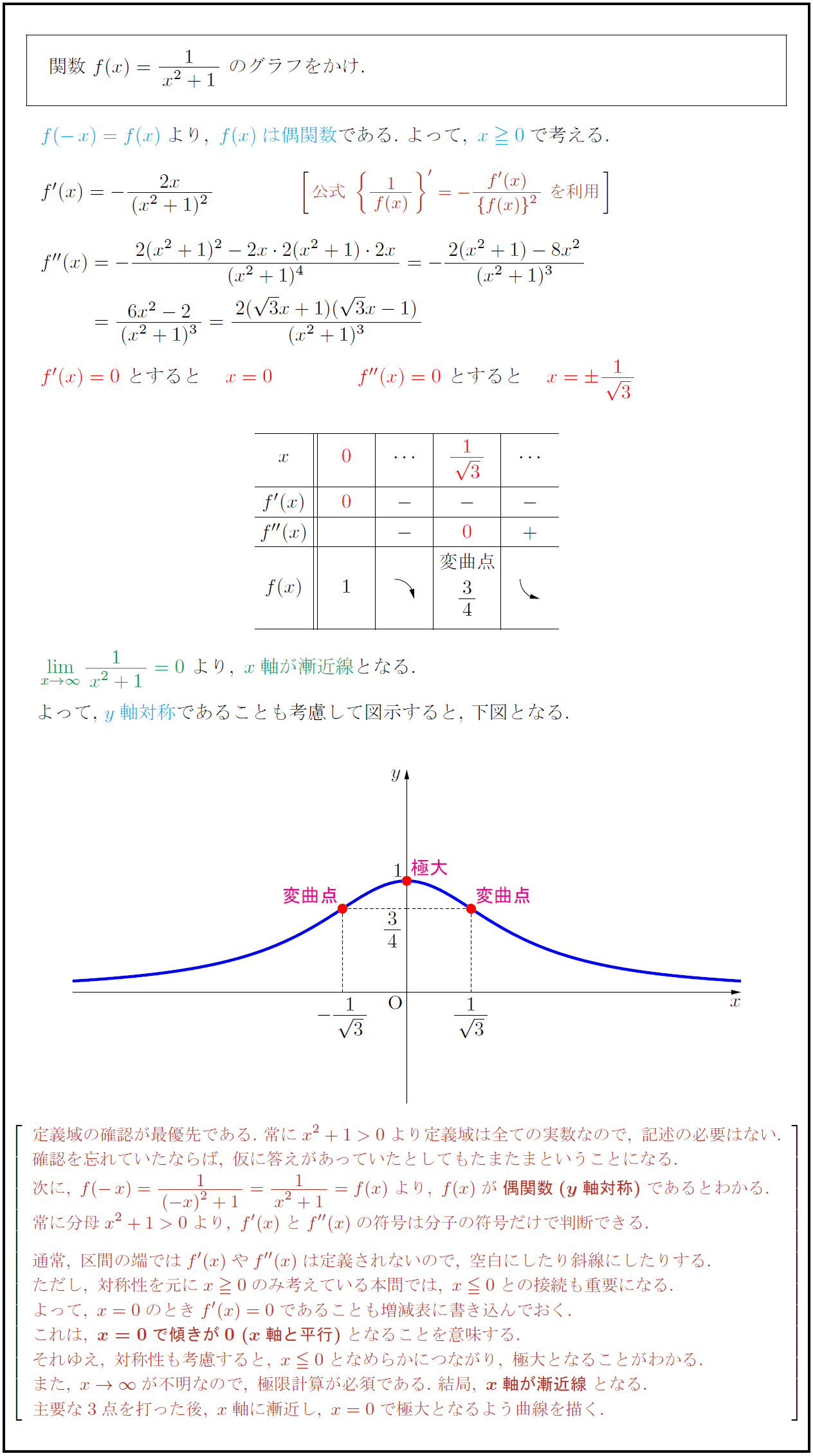
高校数学 分数関数 Y 1 X 1 のグラフ 受験の月

Y X 2 2x 1のグラフあってるでしょうか 解説のグラフと Yahoo 知恵袋

Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history · Parametrisierung einer Kurve a1= y = x^2 auf dem Intervall von 0 bis 2 Gefragt 27 Mai 15 von Gast kurve;Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history

How Do You Graph Y X 2 9 Socratic

高校数学を100倍楽しく No Twitter 数3 微分法とその応用 増減表を書きグラフ を描くのは 慣れるまでは大変です それでは問題です X 2 Y X 2 1のグラフを書いて下さい このグラフが描ければ 勉強も恋愛もうまくいくかも T Co

例題1 y=(x 3) 2 4 のグラフは， y=x 2 のグラフを x 軸の正の向きに 3 ， y 軸の正の向きに 4 だけ平行移動したもので，頂点の座標は (3, 4) ，グラフは右図のようになる．

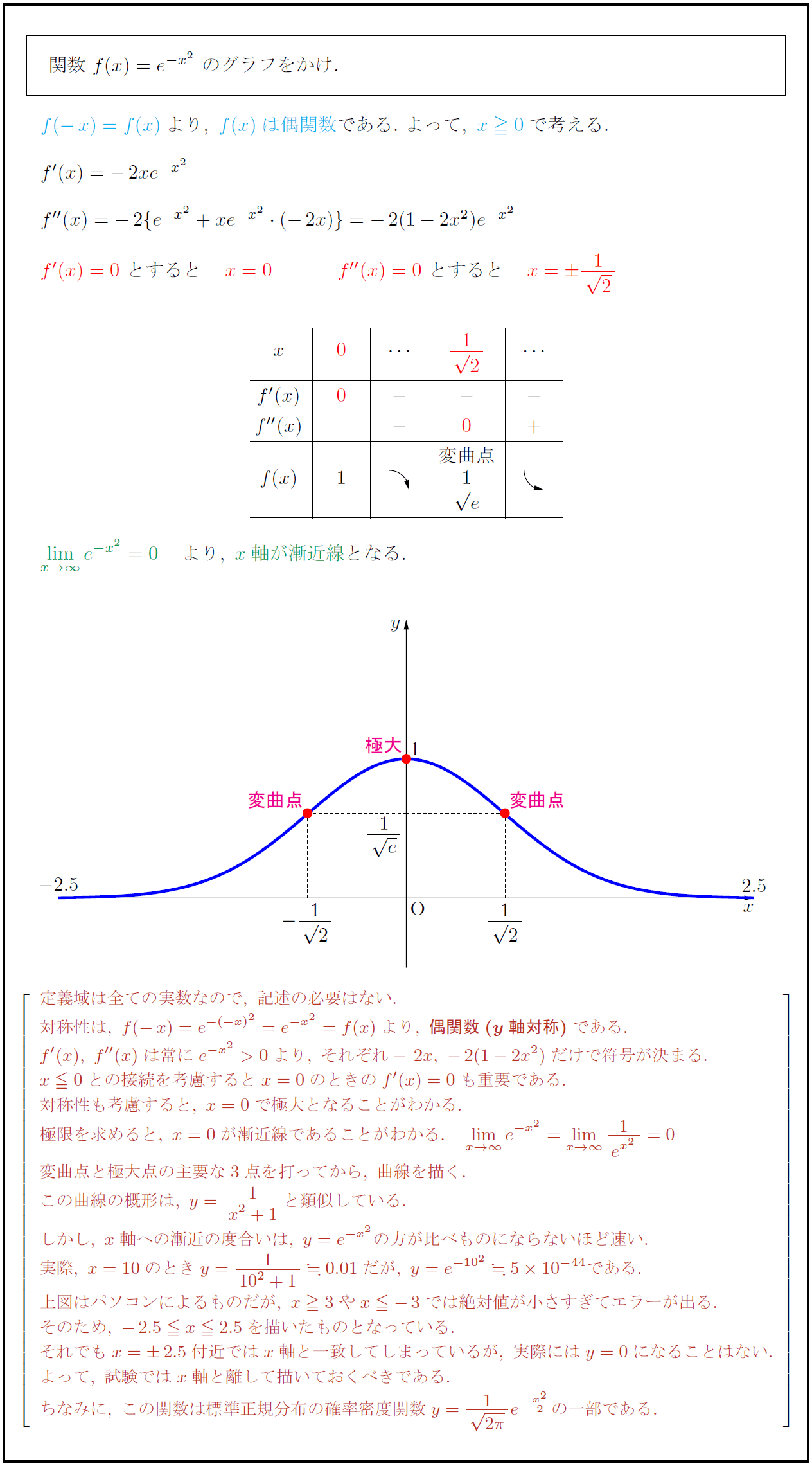
高校数学 指数関数 Y E X のグラフ 正規分布曲線もどき 受験の月

第3回 連続な曲線を描く Memorandom On Julia Language V0 6 4

Y 1 Xの属性 数学についてのwebノート

2x 2 2xy Y 2 4で囲まれた部分の面積を求める問題です Y に直してグラフ Clear

2枚目の教科書の写真から二次関数y 2x 4x 1を2 X 高校数学に関する質問 勉強質問サイト

逆数グラフのイメージ

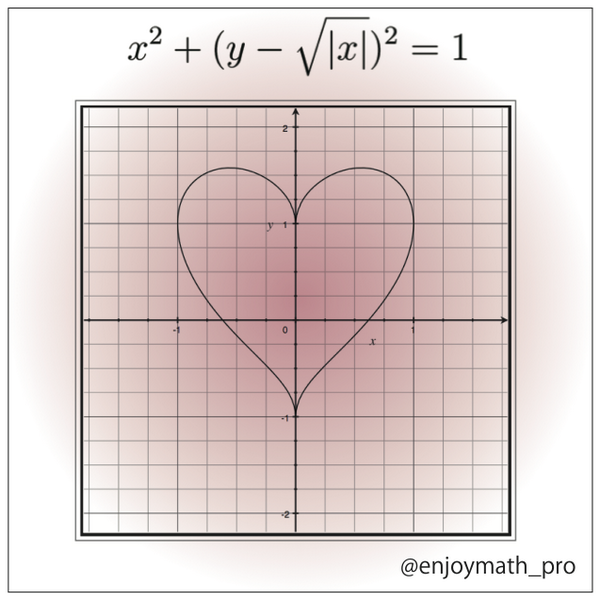
ハートの方程式 大人になってからの再学習

Which Is The Correct Graph Of Arccot X

X 2 Y 3 X 2 2 1のグラフってどうやって解き Yahoo 知恵袋

基礎数学 4回目

偏微分の意味とやり方

1変数関数y F X X 2の性質 数学についてのwebノート

2次曲線のグラフ 微積の授業中に2x 2 4xy 5y 2 6のグラフを書けという 数学 教えて Goo

中学数学 関数

子供向けぬりえ 50 X2 Y21 Graph

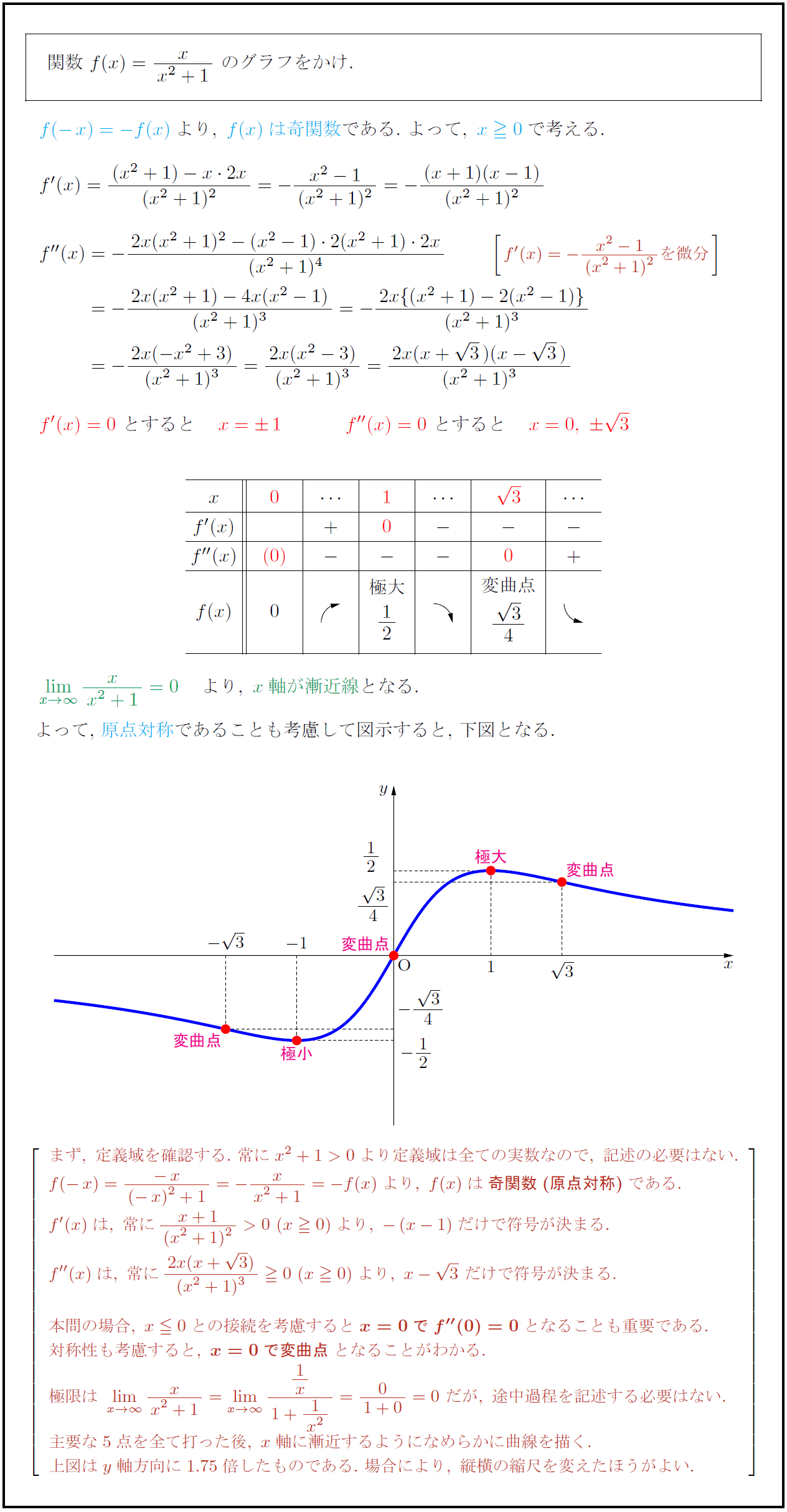
高校数学 分数関数 Y X X 1 のグラフ 受験の月

2変数関数と偏微分 グラフ 接平面を描いてみよう 趣味の大学数学

関数y X 2のグラフ 原点拡大 Geogebra

2次曲線のグラフ 微積の授業中に2x 2 4xy 5y 2 6のグラフを書けという 数学 教えて Goo

212 1 2 がわかりません グラフまでは書けます 高校数学に関する質問 勉強質問サイト

図形のファイル

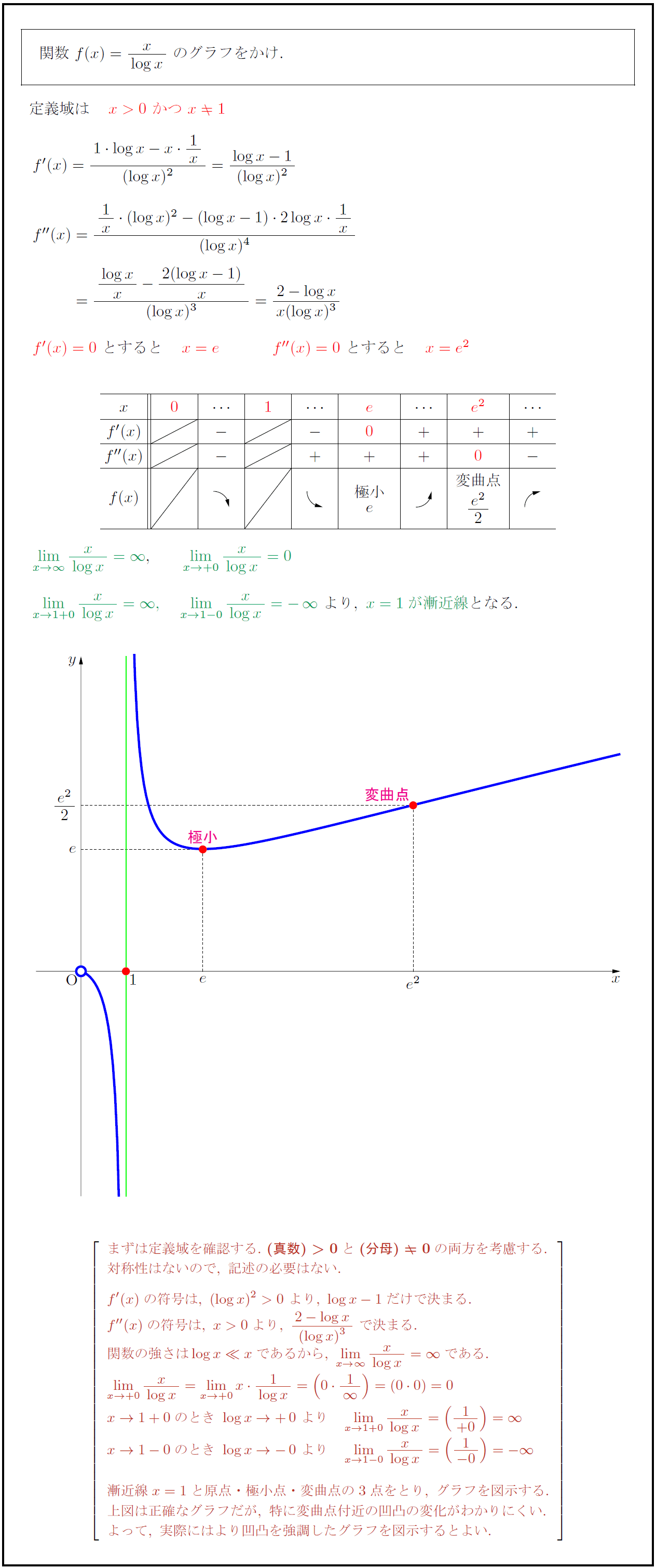
高校数学 対数関数 Y X Logx のグラフ 受験の月

Gaussian Integral Wikipedia

場合分けで答えは と が一緒になっていたのですが 高校数学に関する質問 勉強質問サイト

X 2 Y 2 1 Page 1 Line 17qq Com

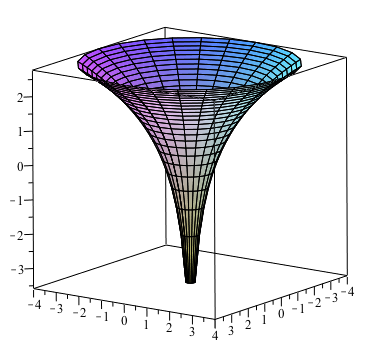
How Do You Sketch F X Y Ln X 2 Y 2 Socratic

Y 3分の1xの2乗の関数のグラフはどのようにしてかけばいいの Yahoo 知恵袋

２乗に比例するグラフ 中学から数学だいすき

Y 1 X2乗のグラフ Youtube

関数ｙ Log 1 ｘ 2 の増減 極値 グラフの凹凸 変曲点を調べよ Yahoo 知恵袋

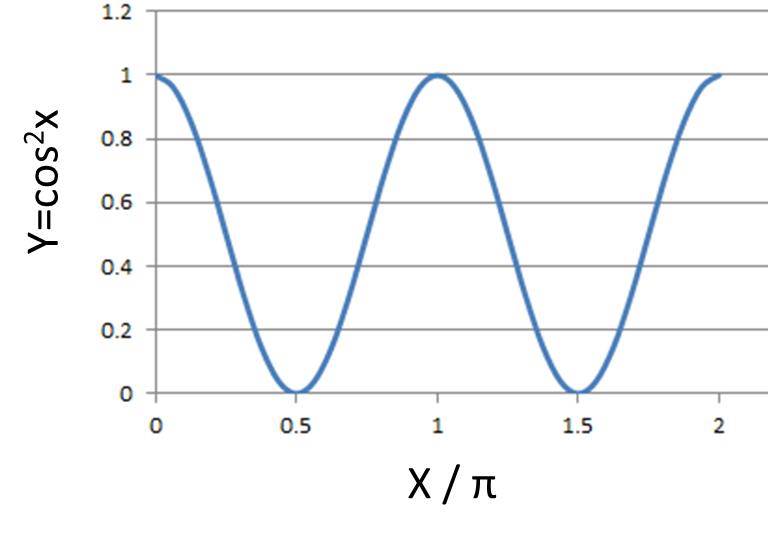
Y Cos 2x のグラフや周期は Y Cos 2 8を微分するとどうなるのか ウルトラフリーダム

1変数関数y F X X 3の性質 数学についてのwebノート

絶対値のついた二次関数のグラフ 高校数学に関する質問 勉強質問サイト

Y 1 1 X 2 Y 1 1 X 2 のグラフの書き方を教えてください また 数学 教えて Goo

数学の微分の応用の問題について質問です 問題 関数 Y X 1 X 2増減 その他 教育 科学 学問 教えて Goo

絶対値のついた二次関数のグラフ 高校数学に関する質問 勉強質問サイト

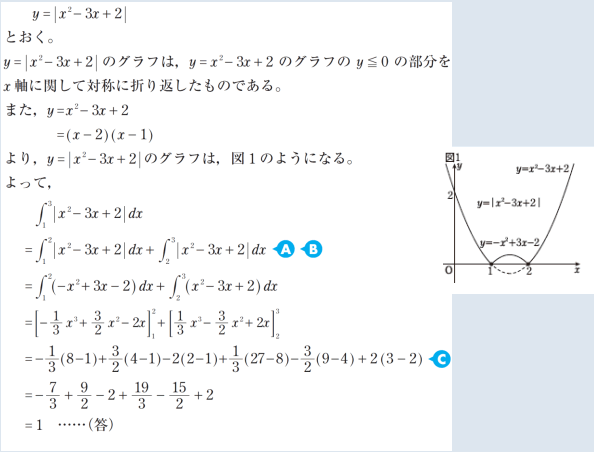
絶対値記号を含む定積分 数学 苦手解決q A 進研ゼミ高校講座

Graph Of Y E X 3 Using Graph Transformations Youtube

質問

基礎数学 5回目

How Do I Graph Z Sqrt X 2 Y 2 1 Without Using Graphing Devices Mathematics Stack Exchange

Graph X 2 Y 2 4 Youtube

How Do You Sketch F X Y Ln X 2 Y 2 Socratic

中学数学 Y Ax 2 のグラフを読み取る 中学数学の無料オンライン学習サイトchu Su

空間の曲面のパラメータ表示 面積

高校数学 グラフのかき方 １ 問題編 映像授業のtry It トライイット

２乗に比例するグラフ 中学から数学だいすき

How To Plot The Graph For The Following Equation Math Sqrt X Sqrt Y 1 Quora

X 2 Y 2 1 Page 1 Line 17qq Com

2次関数についてです ｙ ｘ 2 ａ 1 ｘ 2ｂ ａ ｂは整数 のグラフが 2 ｘ 3の範囲でｘ軸に接するときの問題についてです 高校数学に関する質問 勉強質問サイト

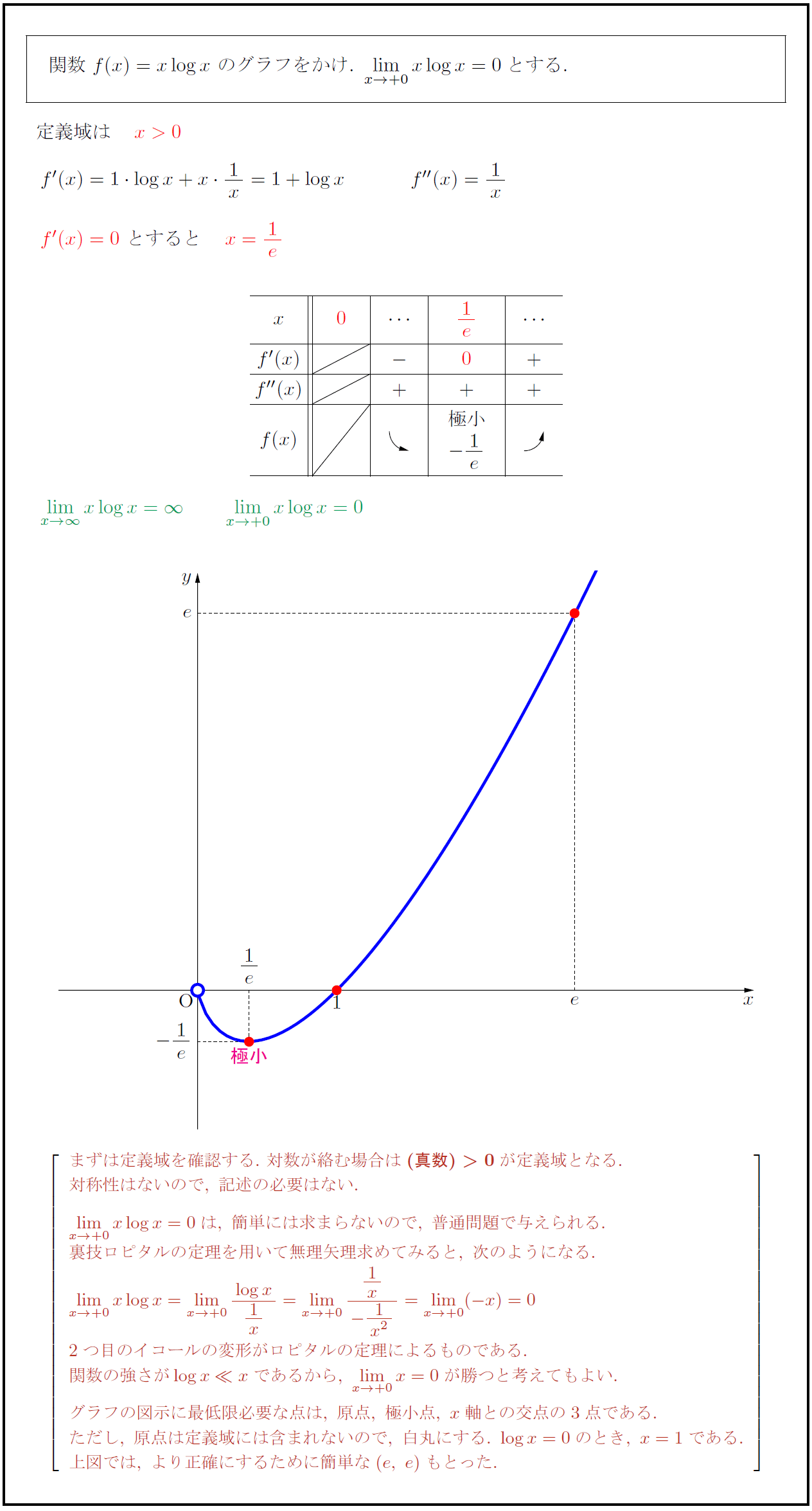
高校数学 対数関数 Y Xlogx のグラフ 受験の月

数式処理ソフト ｍａｘｉｍａ

対数関数のグラフについて

Y Sinxのグラフとその周辺のグラフ 数学ii フリー教材開発コミュニティ Ftext

ルート関数の中身が二次 Y X 2 1 1まで に入ります のグラフはど 数学 教えて Goo

この関数のグラフを描きたいのですが 曲線の式は X 2 Y 3 数学 教えて Goo

基本 二次関数y A X P 2のグラフ なかけんの数学ノート

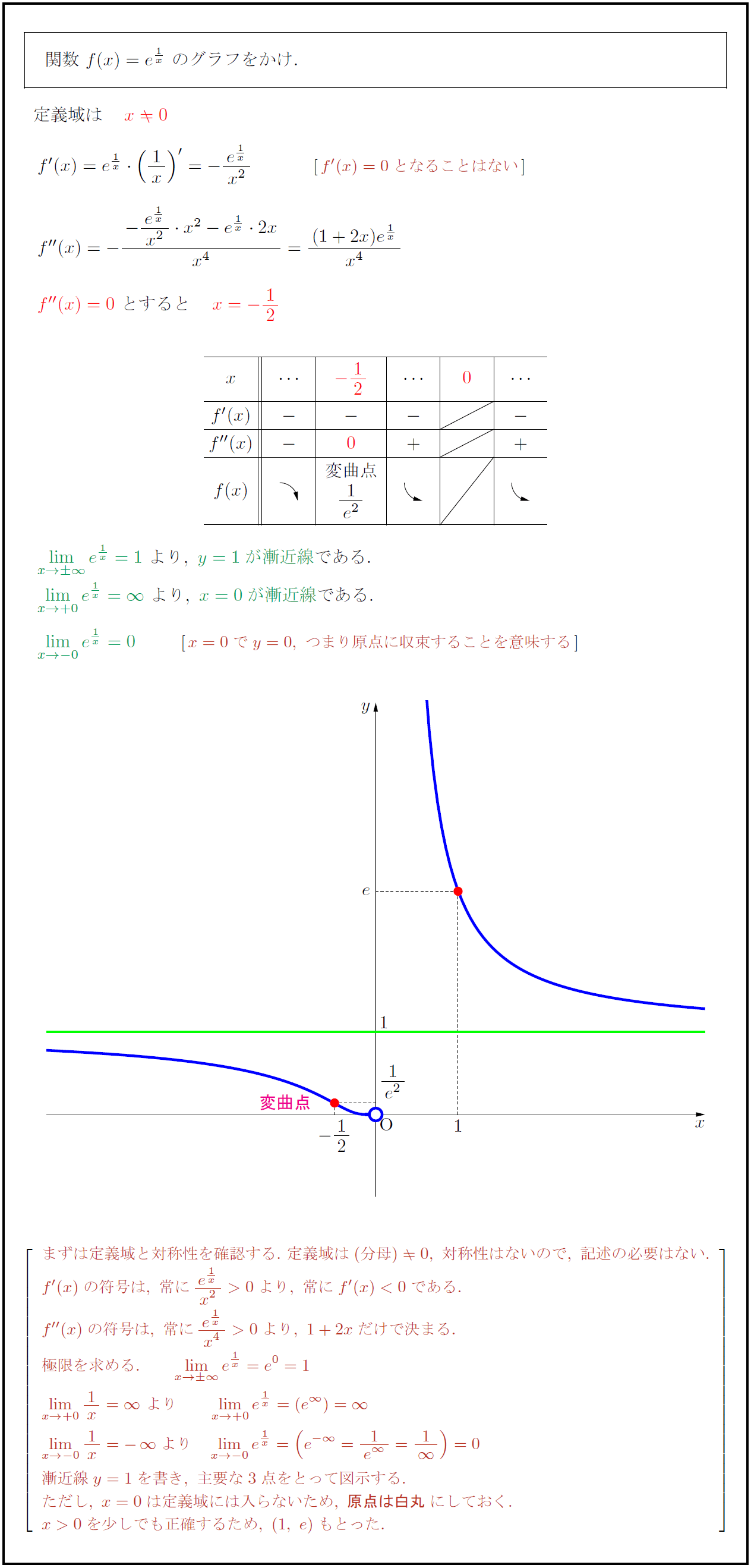
高校数学 指数関数 Y E 1 X のグラフ 受験の月

How To Graph Y 2x 1 Youtube

漸近線の求め方や意味や定義とは 分数関数や双曲線 遊ぶ数学塾

基礎数学 4回目

基本 無理関数のグラフ 放物線の一部 なかけんの数学ノート

逆数グラフのイメージ

子供向けぬりえ 75 Yx21 Graph

美しすぎるハートの数式 プログラムモグモグ

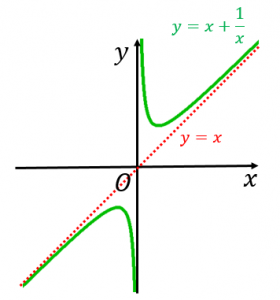
Y X 1 Xの最小値 グラフ 漸近線 具体例で学ぶ数学

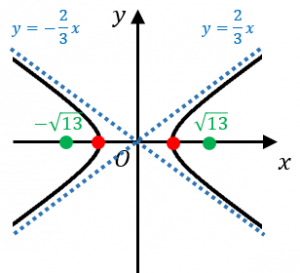
双曲線に関する公式まとめ 具体例で学ぶ数学

子供向けぬりえ 75 Yx21 Graph

基本 無理関数のグラフ 放物線の一部 なかけんの数学ノート

How To Draw A Graph Of Max X Y 1 Quora

X 2 Y 2 1 Page 1 Line 17qq Com

Y Sinxのグラフとその周辺のグラフ 数学ii フリー教材開発コミュニティ Ftext

How Do I Graph Z Sqrt X 2 Y 2 1 Without Using Graphing Devices Mathematics Stack Exchange

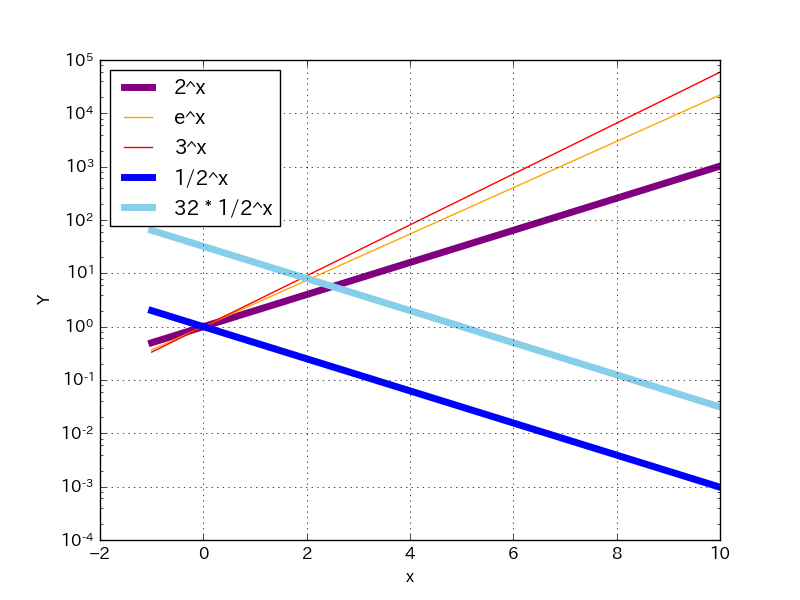
指数と対数 その２ 累乗と累乗根 対数グラフ ふシゼン

Integration Area Enclosed By Graph Of X 4 Y 4 1 Mathematics Stack Exchange

Y X 1 X 2 のグラフをかけという問題なのですが 写真の下線部 Yahoo 知恵袋

How To Graph Y X 2 1 Youtube

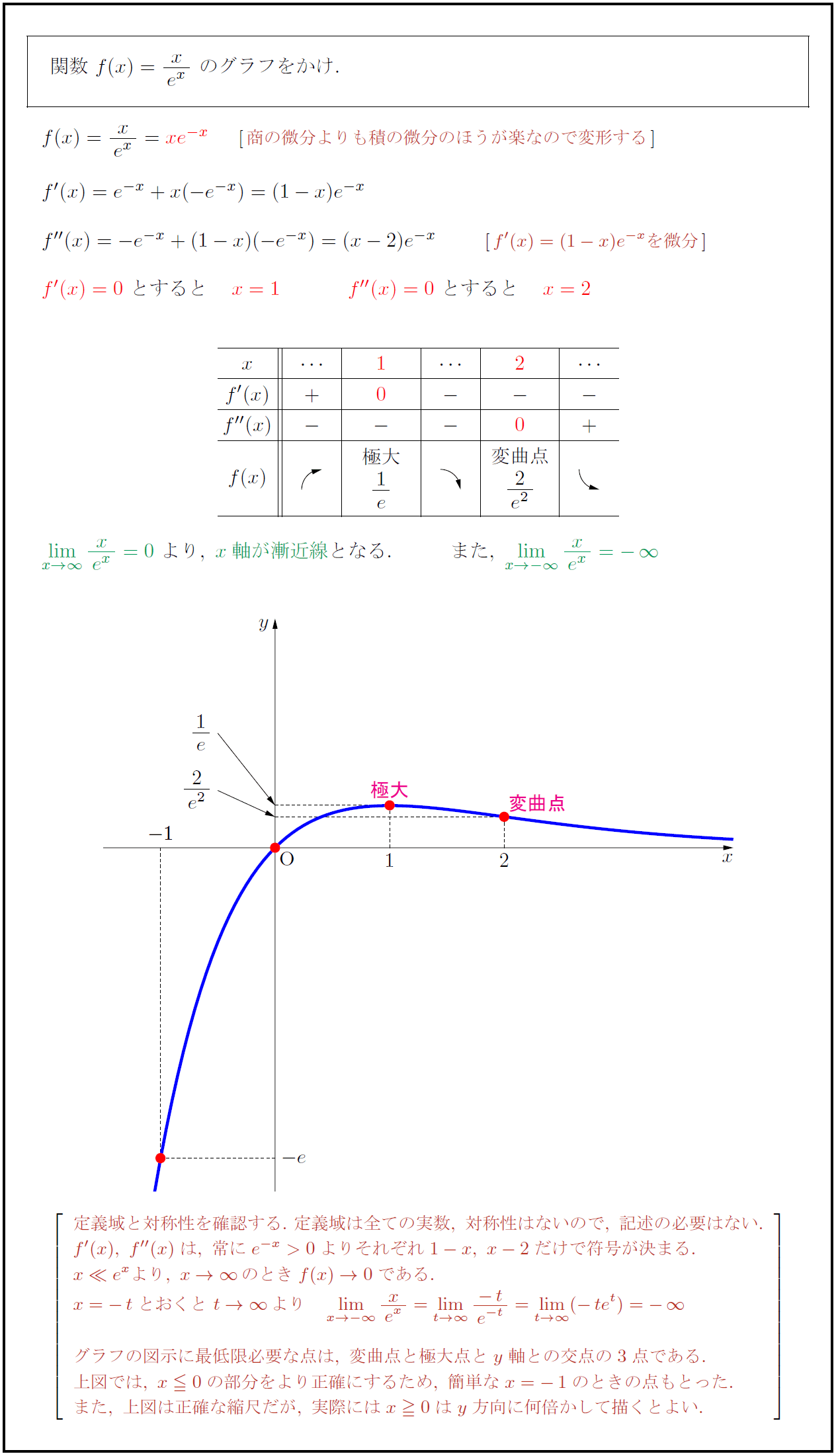
高校数学 指数関数 Y X E X Xe X のグラフ 受験の月

Incoming Term: y=x^2+1 グラフ, y=x^2+1 グラフ 書き方, y=2(x+1)二乗 グラフ, y=2(x-1)2乗 グラフ, y=log(x^2+1) グラフ, y=√(1-x^2) グラフ, y=x^2+2x+1 グラフ, y=1/√1+x^2 グラフ, x^2+xy+y^2=1 グラフ, x^2+y^2+z^2=1 グラフ,